If tan x = 3/2 tan 2(arctan(3/2)) = 12/5 = 24 arctan (3/2) = 5631° 2×5631° = ° tan ° = 12/5 = 24Tan(x y) = (tan x tan y) / (1 tan x tan y) sen(2x) = 2 sen x cos x cos(2x) = cos ^2 (x) sen ^2 (x) = 2 cos ^2 (x) 1 = 1 2 sen ^2 (x) tan(2x) = 2 tan(x) / (1Check https//mathstackexchangecom/q/ Yes, your answer is correct You can continue simplification First of all, you can conclude that (1\tan x)^2= (1\frac {\sin x} {\cos x})^2= (\frac {\sin x\cos x} {\cos x})^2=\frac {12\sin x \cos x} {\cos^2x} Yes, your answer is correct
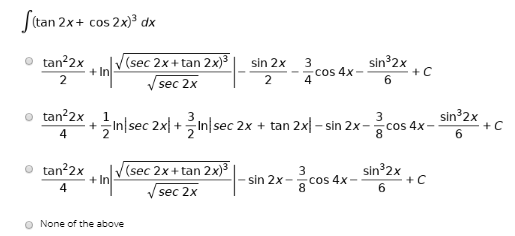
Answered Stan 2x Cos 2x Dx Tan 2x 2 V Sec 2x Bartleby
Tan 2x derivative
Tan 2x derivative-Integreren is een basisbewerking uit de analysengezien integreren niet, zoals bij differentiëren, door eenvoudige regels plaatsvindt, zijn tabellen met veel voorkomende integralen een handig hulpmiddelIn de onderstaande lijst van integralen wordt van een groot aantal verschillende functies de primitieve functie gegeven Er zijn lijsten van integralen2503 · The derivative of tan(2x) can be found by using the quotient rule and the chain rule Using the quotient rule, the tangent of 2x can be simplified to read the cosine squared of 2x plus the sine squared of 2x divided by the cosine squared of 2x Using the Pythagorean identity, this equation further simplifies to one over the cosine squared of 2x



What Is Integral Of Sin2x Tan2x Quora
· I have two problems I am stuck on, if you could show me how to solve the problems it would be much appreciated 1) Find sin 2x, cos 2x, and tan 2x from the given information tan x = − 1/6, cos x > 0 sin 2x = cos 2x = tan 2x = calculus Find complete length of curve r=a sin^3(theta/3) · Don't forget that you have the function as the exponent of the another function! · The equation $\tan x = \tan 2x \tan 4x \tan 8x$ Ask Question Asked today Active today Viewed 6 times 0 $\begingroup$ In the question we
Derive the expression 1 tan^2x Get the answer to this question and access a vast question bank that is tailored for studentsSolution) Given, Adjacent side (A)= 8 cm Opposite side (OTan(3x) in terms of tan(x), write tan(3x) in terms of tan(x), using the angle sum formula and the double angle formulas, simplifying trig identities, trigono
Tan(2x) = 2 tan(x) / (1 tan ^2 (x)) sin ^2 (x) = 1/2 1/2 cos(2x) cos ^2 (x) = 1/2 1/2 cos(2x) sin x sin y = 2 sin( (x y)/2 ) cos( (x y)/2 ) cos x cos y = 2 sin( (x y)/2 ) sin( (x y)/2 ) · 1tan^2x=sec^2x Change to sines and cosines then simplify 1tan^2x=1(sin^2x)/cos^2x =(cos^2xsin^2x)/cos^2x but cos^2xsin^2x=1 we · Example 14 Show that tan 3𝑥 tan 2𝑥 tan 𝑥 = tan 3𝑥 – tan 2𝑥 – tan 𝑥 We know that 3𝑥 = 2𝑥 𝑥 Therefe, tan 3𝑥 = tan(2𝑥 𝑥
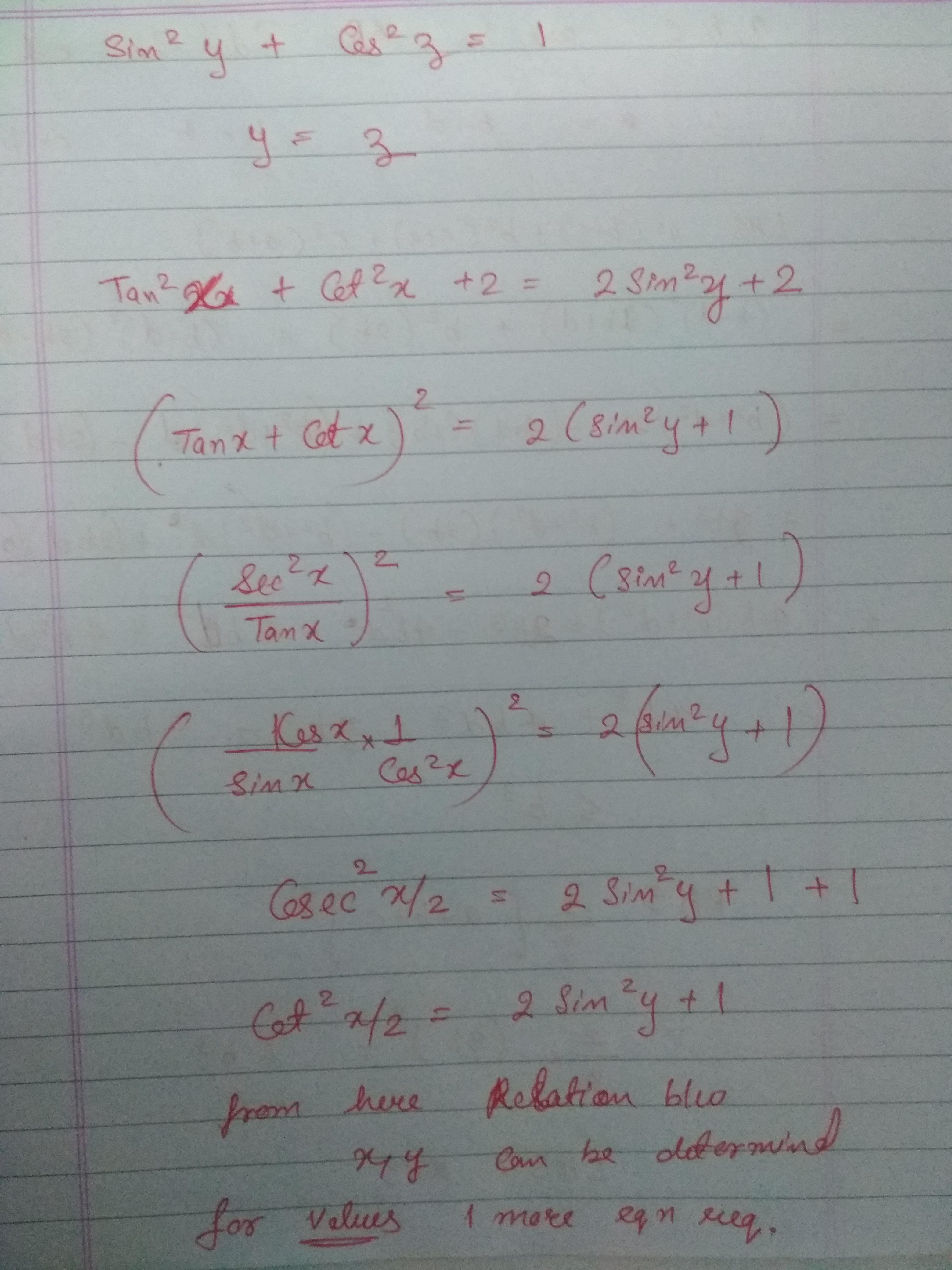


Solve For X Y And Z If Tan 2x Cot 2x 2sin 2y And Sin 2y Cos 2z 1 Askiitians



Prove Tan2x 2tanx 1 Tan 2 X Maths Introduction To Trigonometry Meritnation Com
· Use tan x=sinx/cos x, sin 2x = 2 sin x cos x and cos 2x = cos^2xsin^2x, for the right hand side expression 2 tan x/(1tan^2x)=(2sin x/cos x)/(1(sin^2x/cos^2x) =2 sin x cos x/(cos^2xsin^2x) =(sin 2x)/(cos 2x)=tan 2x Proofs for sin 2x = 2 sin x cos x and cos 2x = 1 2 sin^2x Use Area of a triangleABC = 1/2(base)(altitude) = 1/2 bc sin ASolve for x tan(2x)=1 Take the inverse tangent of both sides of the equation to extract from inside the tangent The exact value of is Divide each term by and simplify Tap for more steps Divide each term in by Cancel the common factor of Tap for more steps Cancel the common factor Divide by Multiply\(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formula \(tan(ab) =\frac{ tan a tan b }{1 tan a tanb}\) So, for this let a = b , it becomes \(tan(aa) =\frac{ tan a tan a }{1 tan a tana}\) \(Tan 2a =\frac{2tan a}{1tan^{2}a} \) Practice Example for tan 2 theta Question Find tan 2 x, if tan x
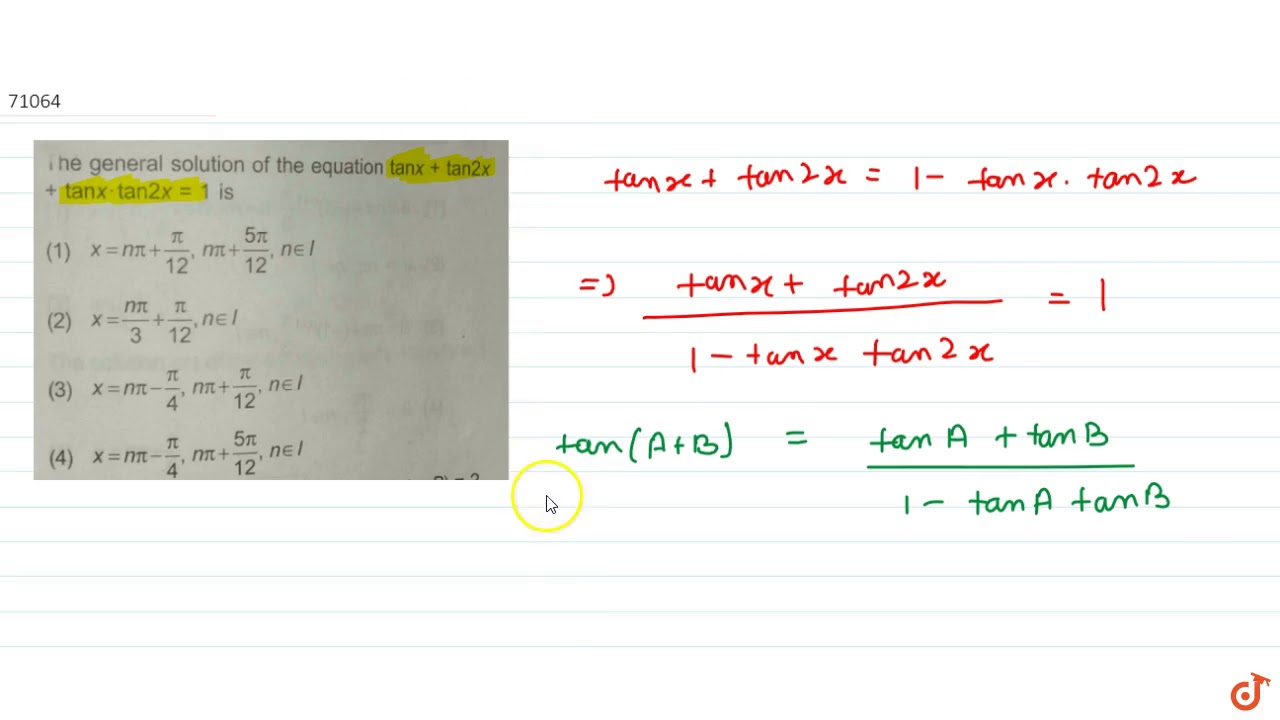


The General Solution Of The Equation Tanx Tan2x Tanx Tan2x 1 Is Youtube



Solve The Following Equation Tan X Tan 2x Tan 3x 0
Sin(x) = sqrt(1cos(x)^2) = tan(x)/sqrt(1tan(x)^2) = 1/sqrt(1cot(x)^2) cos(x) = sqrt(1 sin(x)^2) = 1/sqrt(1tan(x)^2) = cot(x)/sqrt(1cot(x)^2) tan(x) = sin(xIn various applications of trigonometry, it is useful to rewrite the trigonometric functions (such as sine and cosine) in terms of rational functions of a new variable These identities are known collectively as the tangent halfangle formulae because of the definition of These identities can be useful in calculus for converting rational functions in sine and cosine to functions of t in order · In this section we look at how to integrate a variety of products of trigonometric functions These integrals are called trigonometric integralsThey are an important part of the integration technique called trigonometric substitution, which is featured in Trigonometric SubstitutionThis technique allows us to convert algebraic expressions that we may not be able


If Tan X Cot X 2 Find The Value Of Tan 2x Cot 2x
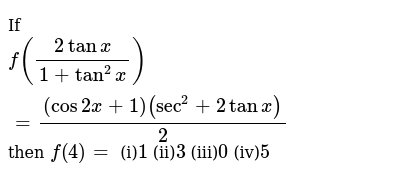


If F 2tanx 1 Tan 2 X Cos2x 1 Sec 2 2tanx 2 Then F 4
Like other substitutions in calculus, trigonometric substitutions provide a method for evaluating an integral by reducing it to a simpler one Trigonometric substitutions take advantage of patterns in the integrand that resemble common trigonometric relations and are most often useful for integrals of radical or rational functions that may not be simply evaluated by other methods0211 · So to find the second derivative of tan(2x), we just need to differentiate 2sec 2 (2x) We can use the chain rule to find the derivative of 2sec 2 (2x) (bearing in mind that the derivative of sec^2(x) is 2sec 2 (x)tan(x)) and it gives us a result of 8sec 2 (2x)tan(2x) The second derivative of tan(2x) is 8sec 2 (2x)tan(2x) · What Is The Integral of cos^2x this process is the reverse of finding a derivative Integrations are the antiderivatives Integrations are the way of


Evaluate Limx 0 X 2 Tan 2x Tan X Sarthaks Econnect Largest Online Education Community


Solved Consider The Possible Identity Tan 2x Cos 2x 1 Cos 2x Sec 2x A State Any Non Permissible Values B Attempt To Verify Possible Identity Course Hero
Graph y=tan(2x) Find the asymptotes Tap for more steps For any , vertical asymptotes occur at , where is an integer Use the basic period for , , to find the vertical asymptotes for Set the inside of the tangent function, , for equal to to find where the vertical asymptote occurs forFree trigonometric equation calculator solve trigonometric equations stepbystep · lim (x tan 2x 2x tan x)/(1 cos 2x)2 (for x → 0) is (a) 2 (b) 2 (c) 1/2 (d) 1/2 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries



1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube
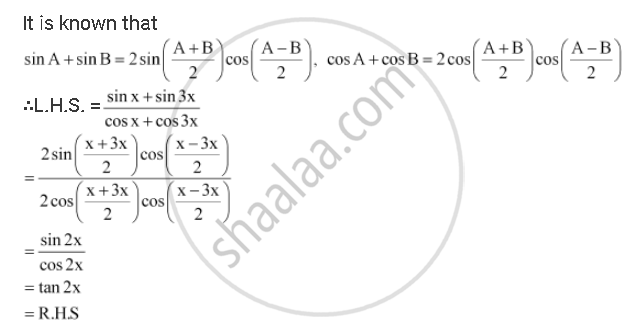


Prove That Sin X Sin 3x Cos X Cos 3x Tan 2x Mathematics Shaalaa Com



0 件のコメント:
コメントを投稿